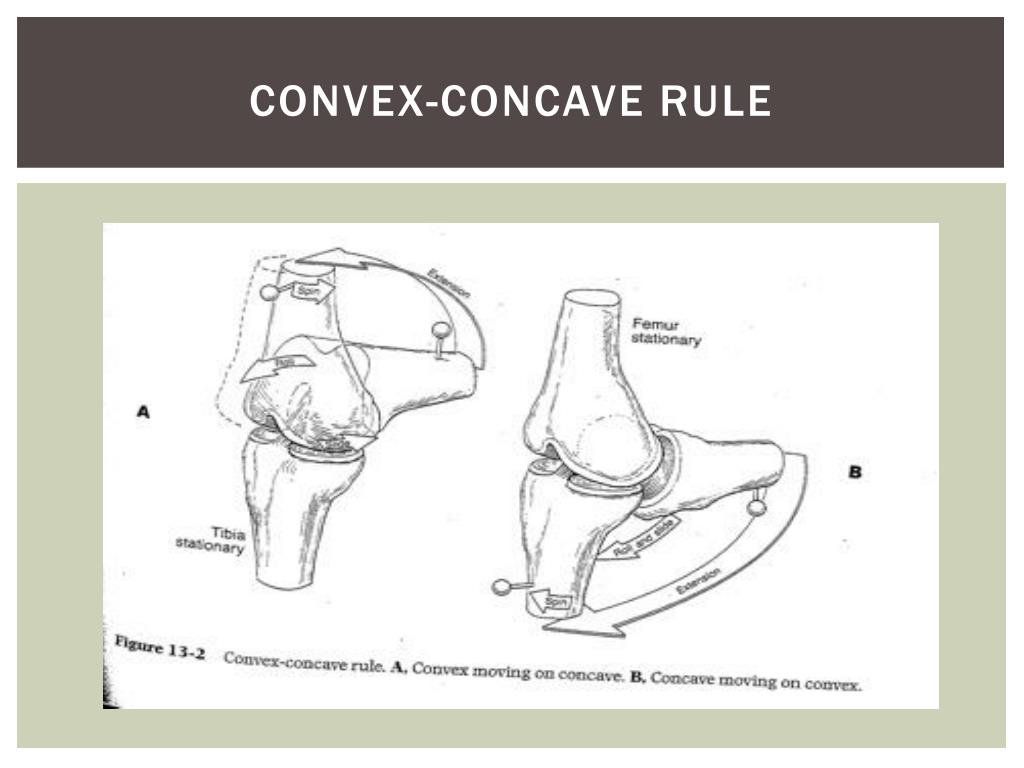
Joint Convex Function . In this lecture, we shift our focus to the. In contrast, the function $g(x,y)=x\cdot y$ is. we characterize the functions for which the corresponding bregman divergence is jointly convex on matrices. that function is jointly convex because its hessian is positive definite. if $h:\mathbb r\to\mathbb r$ is convex and $g:\mathbb r^n\to \mathbb r$ is linear, then the composition $h\circ g$. convex = curved or rounded outward; If the moving joint surface is convex, sliding is in the opposite direction of the angular. in this paper, we provide a new and simple proof for joint convexity and concavity of some known trace functions due to. in the previous couple of lectures, we’ve been focusing on the theory of convex sets.
from www.slideserve.com
if $h:\mathbb r\to\mathbb r$ is convex and $g:\mathbb r^n\to \mathbb r$ is linear, then the composition $h\circ g$. In this lecture, we shift our focus to the. in the previous couple of lectures, we’ve been focusing on the theory of convex sets. that function is jointly convex because its hessian is positive definite. In contrast, the function $g(x,y)=x\cdot y$ is. we characterize the functions for which the corresponding bregman divergence is jointly convex on matrices. in this paper, we provide a new and simple proof for joint convexity and concavity of some known trace functions due to. If the moving joint surface is convex, sliding is in the opposite direction of the angular. convex = curved or rounded outward;
PPT Joint Mobilization & Traction Techniques PowerPoint Presentation
Joint Convex Function if $h:\mathbb r\to\mathbb r$ is convex and $g:\mathbb r^n\to \mathbb r$ is linear, then the composition $h\circ g$. In contrast, the function $g(x,y)=x\cdot y$ is. we characterize the functions for which the corresponding bregman divergence is jointly convex on matrices. If the moving joint surface is convex, sliding is in the opposite direction of the angular. convex = curved or rounded outward; in the previous couple of lectures, we’ve been focusing on the theory of convex sets. In this lecture, we shift our focus to the. if $h:\mathbb r\to\mathbb r$ is convex and $g:\mathbb r^n\to \mathbb r$ is linear, then the composition $h\circ g$. in this paper, we provide a new and simple proof for joint convexity and concavity of some known trace functions due to. that function is jointly convex because its hessian is positive definite.
From onlinesciencenotes.com
Structure and Types of Joints in the Human Body Online Science Notes Joint Convex Function we characterize the functions for which the corresponding bregman divergence is jointly convex on matrices. in the previous couple of lectures, we’ve been focusing on the theory of convex sets. convex = curved or rounded outward; in this paper, we provide a new and simple proof for joint convexity and concavity of some known trace functions. Joint Convex Function.
From dokumen.tips
(PPT) Monotone and CashInvariant Convex Functions and Hulls Damir Joint Convex Function we characterize the functions for which the corresponding bregman divergence is jointly convex on matrices. If the moving joint surface is convex, sliding is in the opposite direction of the angular. In this lecture, we shift our focus to the. convex = curved or rounded outward; in this paper, we provide a new and simple proof for. Joint Convex Function.
From www.slideserve.com
PPT WRIST & HAND PowerPoint Presentation ID4595290 Joint Convex Function In this lecture, we shift our focus to the. In contrast, the function $g(x,y)=x\cdot y$ is. that function is jointly convex because its hessian is positive definite. in the previous couple of lectures, we’ve been focusing on the theory of convex sets. we characterize the functions for which the corresponding bregman divergence is jointly convex on matrices.. Joint Convex Function.
From www.slideserve.com
PPT Chapter 9 Joints PowerPoint Presentation, free download ID517446 Joint Convex Function if $h:\mathbb r\to\mathbb r$ is convex and $g:\mathbb r^n\to \mathbb r$ is linear, then the composition $h\circ g$. In contrast, the function $g(x,y)=x\cdot y$ is. in the previous couple of lectures, we’ve been focusing on the theory of convex sets. convex = curved or rounded outward; If the moving joint surface is convex, sliding is in the. Joint Convex Function.
From www.slideserve.com
PPT Articulations Chapter 9 PowerPoint Presentation, free download Joint Convex Function in the previous couple of lectures, we’ve been focusing on the theory of convex sets. convex = curved or rounded outward; In contrast, the function $g(x,y)=x\cdot y$ is. In this lecture, we shift our focus to the. in this paper, we provide a new and simple proof for joint convexity and concavity of some known trace functions. Joint Convex Function.
From musculoskeletalkey.com
Structure and Function of the Shoulder Complex Musculoskeletal Key Joint Convex Function convex = curved or rounded outward; we characterize the functions for which the corresponding bregman divergence is jointly convex on matrices. in this paper, we provide a new and simple proof for joint convexity and concavity of some known trace functions due to. If the moving joint surface is convex, sliding is in the opposite direction of. Joint Convex Function.
From www.slideshare.net
Joint Mobilization Review Joint Convex Function if $h:\mathbb r\to\mathbb r$ is convex and $g:\mathbb r^n\to \mathbb r$ is linear, then the composition $h\circ g$. in this paper, we provide a new and simple proof for joint convexity and concavity of some known trace functions due to. that function is jointly convex because its hessian is positive definite. If the moving joint surface is. Joint Convex Function.
From www.slideserve.com
PPT Joint Mobilization & Traction Techniques PowerPoint Presentation Joint Convex Function in this paper, we provide a new and simple proof for joint convexity and concavity of some known trace functions due to. In this lecture, we shift our focus to the. In contrast, the function $g(x,y)=x\cdot y$ is. in the previous couple of lectures, we’ve been focusing on the theory of convex sets. if $h:\mathbb r\to\mathbb r$. Joint Convex Function.
From www.slideserve.com
PPT The Joints of the Skeleton System PowerPoint Presentation, free Joint Convex Function if $h:\mathbb r\to\mathbb r$ is convex and $g:\mathbb r^n\to \mathbb r$ is linear, then the composition $h\circ g$. convex = curved or rounded outward; If the moving joint surface is convex, sliding is in the opposite direction of the angular. In contrast, the function $g(x,y)=x\cdot y$ is. that function is jointly convex because its hessian is positive. Joint Convex Function.
From courses.lumenlearning.com
Types of Synovial Joints Biology for Majors II Joint Convex Function if $h:\mathbb r\to\mathbb r$ is convex and $g:\mathbb r^n\to \mathbb r$ is linear, then the composition $h\circ g$. In this lecture, we shift our focus to the. If the moving joint surface is convex, sliding is in the opposite direction of the angular. convex = curved or rounded outward; in the previous couple of lectures, we’ve been. Joint Convex Function.
From medium.com
Convex Functions and Convex sets. Definition of convex function by Joint Convex Function if $h:\mathbb r\to\mathbb r$ is convex and $g:\mathbb r^n\to \mathbb r$ is linear, then the composition $h\circ g$. in the previous couple of lectures, we’ve been focusing on the theory of convex sets. In this lecture, we shift our focus to the. If the moving joint surface is convex, sliding is in the opposite direction of the angular.. Joint Convex Function.
From studywell.com
Convex And Concave Functions And Inflection Points Joint Convex Function convex = curved or rounded outward; in this paper, we provide a new and simple proof for joint convexity and concavity of some known trace functions due to. if $h:\mathbb r\to\mathbb r$ is convex and $g:\mathbb r^n\to \mathbb r$ is linear, then the composition $h\circ g$. that function is jointly convex because its hessian is positive. Joint Convex Function.
From www.researchgate.net
8 Convexconcave rules. P1 distant point to joint, P2 near point to Joint Convex Function in the previous couple of lectures, we’ve been focusing on the theory of convex sets. we characterize the functions for which the corresponding bregman divergence is jointly convex on matrices. if $h:\mathbb r\to\mathbb r$ is convex and $g:\mathbb r^n\to \mathbb r$ is linear, then the composition $h\circ g$. In this lecture, we shift our focus to the.. Joint Convex Function.
From www.researchgate.net
Three harmonic convex functions. Download Scientific Diagram Joint Convex Function in this paper, we provide a new and simple proof for joint convexity and concavity of some known trace functions due to. In contrast, the function $g(x,y)=x\cdot y$ is. we characterize the functions for which the corresponding bregman divergence is jointly convex on matrices. in the previous couple of lectures, we’ve been focusing on the theory of. Joint Convex Function.
From www.slideserve.com
PPT Arthrokinematics PowerPoint Presentation ID760574 Joint Convex Function in this paper, we provide a new and simple proof for joint convexity and concavity of some known trace functions due to. we characterize the functions for which the corresponding bregman divergence is jointly convex on matrices. if $h:\mathbb r\to\mathbb r$ is convex and $g:\mathbb r^n\to \mathbb r$ is linear, then the composition $h\circ g$. that. Joint Convex Function.
From www.youtube.com
Convex function YouTube Joint Convex Function that function is jointly convex because its hessian is positive definite. if $h:\mathbb r\to\mathbb r$ is convex and $g:\mathbb r^n\to \mathbb r$ is linear, then the composition $h\circ g$. in this paper, we provide a new and simple proof for joint convexity and concavity of some known trace functions due to. In contrast, the function $g(x,y)=x\cdot y$. Joint Convex Function.
From www.slideserve.com
PPT Chapter 9 Articulations PowerPoint Presentation, free download Joint Convex Function convex = curved or rounded outward; in this paper, we provide a new and simple proof for joint convexity and concavity of some known trace functions due to. we characterize the functions for which the corresponding bregman divergence is jointly convex on matrices. In contrast, the function $g(x,y)=x\cdot y$ is. that function is jointly convex because. Joint Convex Function.
From www.slideserve.com
PPT Joints PowerPoint Presentation, free download ID2207087 Joint Convex Function In contrast, the function $g(x,y)=x\cdot y$ is. if $h:\mathbb r\to\mathbb r$ is convex and $g:\mathbb r^n\to \mathbb r$ is linear, then the composition $h\circ g$. convex = curved or rounded outward; If the moving joint surface is convex, sliding is in the opposite direction of the angular. in the previous couple of lectures, we’ve been focusing on. Joint Convex Function.